Being able to find the Greatest Common Factor of two numbers is an important basic foundational skill. It goes WAY back to what you learned in elementary school…and the days of the 'greatest common factor' and 'greatest common divisor.' Let's see how you can put that old knowledge to use in Pre-algebra!

So, let's say you have two monomials like

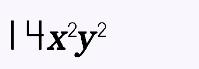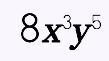and, you wanted to find out what the Greatest Common Factor they share is. What would be the best or easiest method for finding it? Check this out!

Step 1: Write out the monomials side-by-side.

Write the monomials side-by-side, horizontally. Then, draw an upside-down division 'house' as shown.

Step 2: Look at the COEFFICIENTS.

Starting with the coefficients, see if you can find a common factor/divisor.

IF they share a common factor/divisor, then use it!

Write this number on the outside of the 'house,' as shown. Write the dividend below.

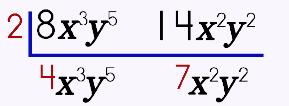Step 3: Look at the first common variable.

Next, look at the first common variable. Write this variable on the outside of the 'house,' as shown.

Factor it, including the number of EXPONENTS (remember to use the exponent rules!).

Re-write the dividend below.

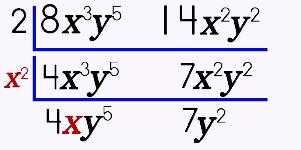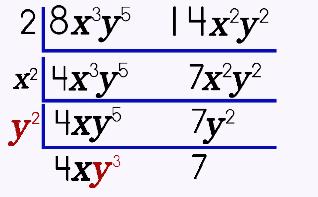Step 4: Continue factoring the variables. Each variable/step has its own floor on the house!

Continue looking for COMMON VARIABLES. Factor them out.

First, write the common variable on the outside of the 'house.' Then, write the new dividend at the bottom of the 'house.'

Make a new 'house' each time. This way, you won't make an error!

 

*Each new step should have add a new floor to the house!

Step 5: Writing the GCF…

The Greatest Common Factor (or Greatest Common Divisor) is now easy to find.

 

Look along the LEFT side of the house. Multiply each of these terms together, and voila!

You have now found the common factors of the two polynomials.

 

Easy-peasy, lemon-squeasy!

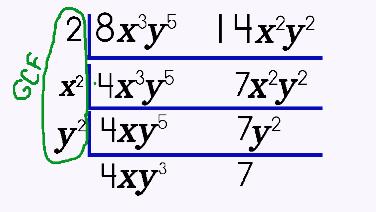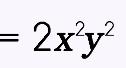©2011–2017 Sherry Skipper Spurgeon. All rights reserved.